

Next: About this document ...
Up: Quiz1
Previous: Exercise 2.
Let p, q two different prime integers.
Let a be another integer, not necessarily prime
and such that a 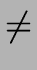 0 and neither p nor q
divides a.
0 and neither p nor q
divides a.
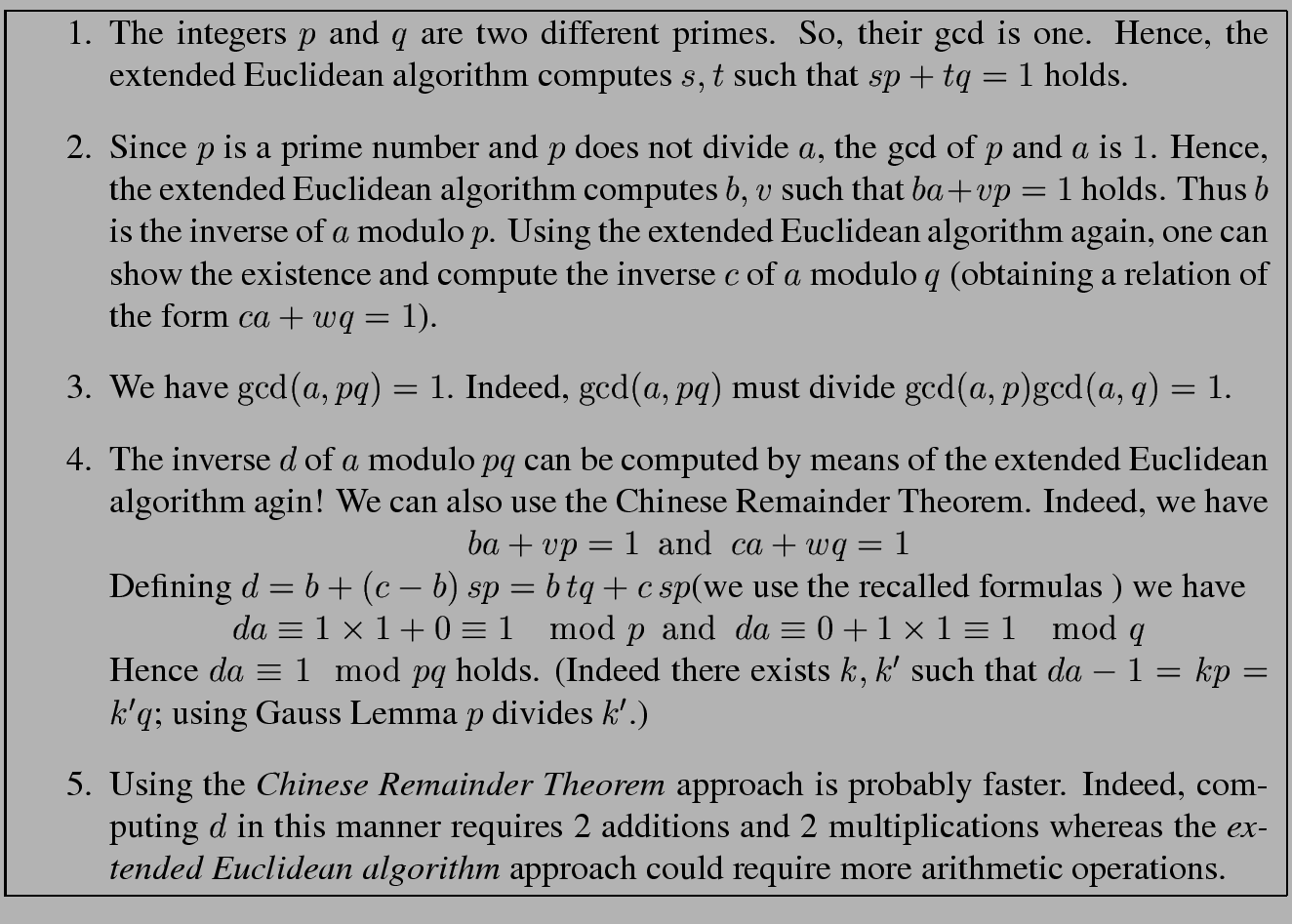
- Explain briefly why there exists two integers s, t
such that
sp + tq = 1 holds.
How can we compute them?
- Explain briefly why a has an inverse b modulo p
and an inverse c modulo q.
How can we compute them?
- Explain briefly why a has an inverse d modulo pq.
- Assume that
s, t, b, c have been computed.
Explain briefly at least two ways to compute d.
- Which way do you feel to be the best one?
Answer 4


Next: About this document ...
Up: Quiz1
Previous: Exercise 2.
Marc Moreno Maza
2006-01-09
