Exercise 1.

In this exercise, detailed and precise explanations are required. As expected, we consider the field 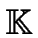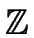$\mbox{${\mathbb K}$}$ = $\mbox{${\mathbb Z}$}$/17$\mbox{${\mathbb Z}$}$.

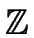1. Show that 3 is in 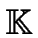$\mathbb {K}$ a primitive 16-th root of unity.
2. Find all primitive 4-th roots of unity in $\mathbb {K}$.

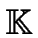Answer 1  

Answer 2