Definition 4
Let (
G,.) be a (multiplicative) group with neutral element
e.
A nonempty subset
H 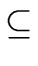 G
G is a
subgroup of
G
if the following three statements hold
- e
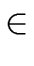 H,
H,
- for every
x, y
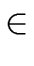 H we have
x y
H we have
x y 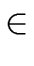 H,
H,
- for every x
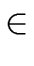 H the inverse x-1 of x belongs to H.
H the inverse x-1 of x belongs to H.
Theorem 3
For every subgroup
H of the additive abelian group
(
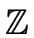
, +)
there exists an element
a 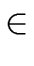
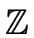
such that
H is the set
of the multiples of
a, that is
H =
a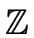
.
Theorem 4
Let
G be a multiplicative group with neutral element
e.
Let
x 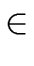 G
G an element and
gr(
x)
the subgroup of
H consisting of all powers of
x (including
e =
x0 and
x-1 the inverse of
x).
Let

(
x) be the order of
gr(
x),
that is the cardinality of
gr(
x).
Then two cases arise
- either gr(x) is infinite and then the powers
of x are pairwise different and thus H is isomorphic to
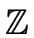 .
.
- or gr(x) is finite and we have the following properties
-
 (x) is the smallest integer n such that xn = e,
(x) is the smallest integer n such that xn = e,
-
xm = xm' iff
m
 m'mod
m'mod  (x),
(x),
- H is isomorphic to
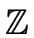 /n
/n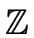 where
n =
where
n =  (x),
(x),
-
H = {e, x, x2,..., xn-1} where
n =
 (x).
(x).
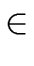 H,
H,
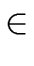 H we have
x y
H we have
x y 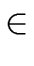 H,
H,
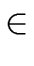 H the inverse x-1 of x belongs to H.
H the inverse x-1 of x belongs to H.